Answers
Answer:
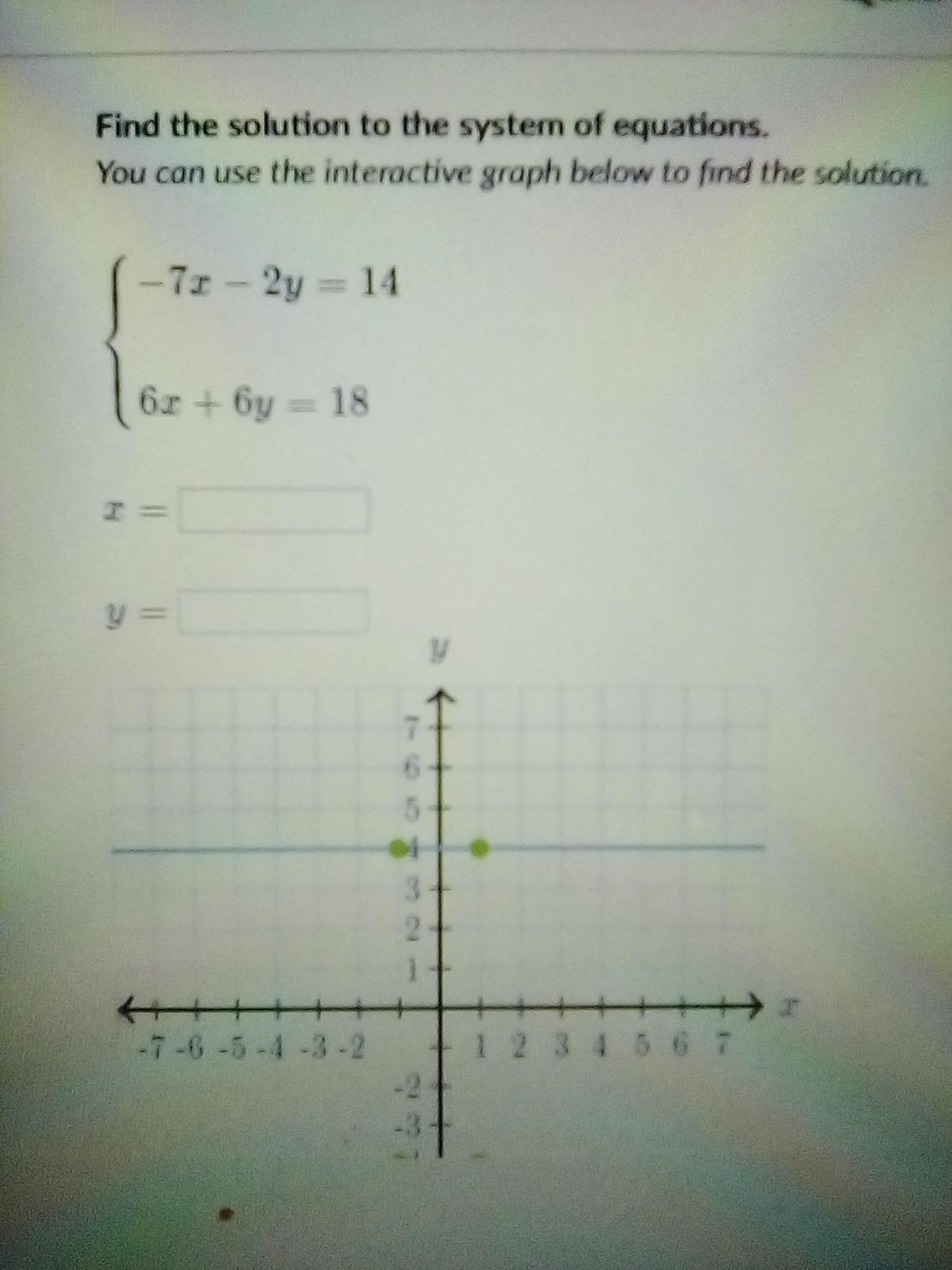
x = - 4, y = 7
Step-by-step explanation:
Given the 2 equations
- 7x - 2y = 14 → (1)
6x + 6y = 18 → (2)
Multiplying (1) by 3 and adding to (2) will eliminate the y- term
- 21x - 6y = 42 → (3)
Add (2) and (3) term by term to eliminate y
- 15x = 60 ( divide both sides by 15 )
x = - 4
Substitute x = - 4 into either of the 2 equations and evaluate for y
Substituting into (2)
6(- 4) + 6y = 18
- 24 + 6y = 18 ( add 24 to both sides )
6y = 42 ( divide both sides by 6 )
y = 7
Related Questions
need some help thxx ;)
Answers
Answer:
DEA
Step-by-step explanation:
6th grade math , help me please :)
Answers
Answer:B
Step-by-step explanation:
A facilities manager at a university reads in a research report that the mean amount of time spent in the shower by an adult is 5 minutes. He decides to collect data to see if the mean amount of time that college students spend in the shower is significantly different from 5 minutes. In a sample of 11 students, he found the average time was 4.52 minutes and the standard deviation was 0.75 minutes. Using this sample information, conduct the appropriate hypothesis test at the 0.1 level of significance. Assume normality. (can you please show how to do this without a calculator or excel i just dont want answer but want to know how to do it).
a) What are the appropriate null and alternative hypotheses?
A) H0: μ = 5 versus Ha: μ < 5
B) H0: μ = 5 versus Ha: μ ≠ 5
C) H0: x = 5 versus Ha: x ≠ 5
D) H0: μ = 5 versus Ha: μ > 5
b) What is the test statistic? Give your answer to four decimal places.
c) What is the P-value for the test? Give your answer to four decimal places.
d) What is the appropriate conclusion?
A) Fail to reject the claim that the mean time is 5 minutes because the P-value is larger than 0.01.
B) Reject the claim that the mean time is 5 minutes because the P-value is larger than 0.01.
C) Reject the claim that the mean time is 5 minutes because the P-value is smaller than 0.01.
D) Fail to reject the claim that the mean time is 5 minutes because the P-value is smaller than 0.01.
Answers
Answer:
A) Null Hypothesis;H0: μ = 5
Alternative Hypothesis;Ha: μ ≠ 5
B) test statistic = -2.1226
C) p-value = 0.0598
D) Option A is correct
Step-by-step explanation:
We are given;
x = 4.52 minutes
s = 0.75 minutes
μ = 5 minutes
n = 11
degree of freedom = n - 1 = 11 - 1 = 10
A) The hypotheses are;
Null Hypothesis;H0: μ = 5
Alternative Hypothesis;Ha: μ ≠ 5
B) t-statistic = (x - μ)/(s/√n)
(4.52 - 5)/(0.75/√11) = -2.1226
C) From the t-score calculator results attached, the p-value is approximately 0.0598.
D) The P-value of 0.0598 is is greater than the significance level of 0.01, thus we fail to reject the null hypothesis, and we say that the result is statistically nonsignificant. So option A is correct.
Con proceso por favor
Answers
Answer:se
Step-by-step explanation:
Six identical coins are tossed. How many possible arrangements of the coins include three heads and three tails?
Answers
Answer:
The possible arrangement= 18 ways
Step-by-step explanation:
Six identical coin are tossed.
Coin has only a tail and a head.
In how many possible ways can the arrangement be 3 head and 3 tail.
The possible arrangement= (3! * 3!)/2
The reason for dividing by two because coin has two face.
The possible arrangement= (3! * 3!)/2
The possible arrangement=( 6*6)/2
The possible arrangement= 36/2
The possible arrangement= 18 ways
rectangular field has a total perimeter of 128 feet. The width is
A
24 feet less than the length. What are the dimensions of the field?
Answers
Answer:
The length is 44 feetThe width is 20 feetStep-by-step explanation:
Perimeter of a rectangle = 2l + 2w
where
l is the length
w is the width
Perimeter = 128 feet
The width is 24 feet less than the length is written as
w = l - 24
128 = 2l + 2( l - 24)
128 = 2l + 2l - 48
Group like terms
4l = 176
Divide both sides by 4
l = 44
The length is 44 feet
Substitute l = 44 into w = l - 24
w = 44 - 24
w = 20
The width is 20 feet
Hope this helps you
Find the critical numbers of the function. (Enter your answers as a comma-separated list. If an answer does not exist, enter DNE.) f(x) = x3 + 3x2 − 72x
Answers
Answer:
x = -6 and x = 4
Step-by-step explanation:
In math, the critical points of a function are the points where the derivative equals zero.
So, first we will find the derivative of the function. The derivative is:
[tex]f'(x)=3x^2 +6x-72[/tex]
Now, we are going to make the derivative equal zero and find the answers of the equation.
[tex]3x^2 +6x-72=0\\3(x^2 +2x-24)=0\\3(x+6)(x-4)=0\\[/tex]
So we have that the critical points are the answers to this equation:
[tex]x+6= 0 \\x= - 6[/tex]
and
[tex]x-4=0\\x=4[/tex]
Thus, the critical points are x=-6 and x=4
Using it's concept, it is found that the critical numbers of the function are:
x = -6 and x = 4.
The critical numbers of a function f(x) are the values of x for which it's derivative is zero, that is:
[tex]f^{\prime}(x) = 0[/tex]
In this problem, the function is:
[tex]f(x) = x^3 + 3x^2 - 72x[/tex]
The derivative is:
[tex]f^{\prime}(x) = 3x^2 + 6x - 72[/tex]
[tex]f^{\prime}(x) = 3(x^2 + 2x - 24)[/tex]
Then:
[tex]f^{\prime}(x) = 0[/tex]
[tex]3(x^2 + 2x - 24) = 0[/tex]
[tex]x^2 + 2x - 24 = 0[/tex]
Which is a quadratic equation with coefficients [tex]a = 1, b = 2, c = -24[/tex], which we have to solve.
[tex]\Delta = 2^2 - 4(1)(-24) = 100[/tex]
[tex]x_{1} = \frac{-2 + \sqrt{100}}{2} = 4[/tex]
[tex]x_{2} = \frac{-2 - \sqrt{100}}{2} = -6[/tex]
The critical numbers of the function are x = 4 and x = -6.
A similar problem is given at https://brainly.com/question/16944025
Cameron sponsor a fun raiser that 582 people attended he raise $6480 he charged $15 for balcony seats and $10 for crown seats how many people bought balcony seats and how many people bought ground seats
Answers
Answer:
132 people bought balcony tickets and 450 bout ground tickets
Step-by-step explanation:
let x be balcony and y for ground
x+y=582
15x+10y=6480 solve by adding(subtracting)/eliminating process
multiply first equation by 15 to eliminate x:
15x+15y=8730
15x+10y=6480 subtract
15x+15y-15x-10y=8730-6480
5y=2250
y=2250/5=450
x+y=582
x=582-450=132
Integrate the following: ∫[tex]5x^4dx[/tex]
A. [tex]x^5+C[/tex]
B. [tex]x^5[/tex]
C. [tex]5x^5+C[/tex]
D. [tex]5x^5[/tex]
Answers
Answer:
A. [tex]x^5+C[/tex]
Step-by-step explanation:
This is a great question! The first thing we want to do here is to take the constant out of the expression, in this case 5. Doing so we would receive the following expression -
[tex]5\cdot \int \:x^4dx[/tex]
We can then apply the power rule " [tex]\int x^adx=\frac{x^{a+1}}{a+1}[/tex] ", where a = exponent ( in this case 4 ),
[tex]5\cdot \frac{x^{4+1}}{4+1}[/tex]
From now onward just simplify the expression as one would normally, and afterward add a constant ( C ) to the solution -
[tex]5\cdot \frac{x^{4+1}}{4+1}\\[/tex] - Add the exponents,
[tex]5\cdot \frac{x^{5}}{5}[/tex] - 5 & 5 cancel each other out,
[tex]x^5[/tex] - And now adding the constant we see that our solution is option a!
Answer:
Answer A
Step-by-step explanation:
Use the property of integrals. You now have [tex]5 x\int\limits\,x^{4}dx[/tex] where the first x next to the 5 stands for multiplication. Let's evaluate it. We get [tex]5 (\frac{x^{5} }{5})[/tex]. From here, we can simplify this into [tex]x^{5}[/tex]. Add the constant of integration, which will give you the answer of [tex]x^{5} + C[/tex].
A hospital found that a lower outside temperature indicates a higher number of patient visits. What can we determine from this
Information?
Answers
Answer:
Second Answer
Step-by-step explanation:
Please tell me if I'm right or wrong! No work needed! Brainliest will be given!
Answers
Answer:
The first one is correct
The second one is also correct
The third is also correct
Congrats!
Answer:
1) [tex]\boxed{Option \ B}[/tex]
2) [tex]\boxed{Option \ B}[/tex]
3) [tex]\boxed{Option \ B}[/tex]
You're totally correct, Man! :)
Step-by-step explanation:
Question 1:
[tex](6b^2-4b+3)-(9b^2-3b+6)\\Resolving \ the\ brackets\\6b^2-4b+3-9b^2+3b-6\\Combining \ like \ terms\\6b^2-9b^2-4b+3b+3-6\\-3b^3-b-3[/tex]
Question 2:
[tex](b+6)(b-3)\\Using \ FOIL\\b^2-3b+6b-18\\b^2+3b-18[/tex]
Question 3:
[tex](4x-3)(6x-1)\\Using \ FOIL\\24x^2-4x-18x+3\\24x^2-22x+3[/tex]
Factor the polynomial completely.
Q(x) = x4 − 1
Q(x)=
Find all its zeros. State the multiplicity of each zero. (Order your answers from smallest to largest real, followed by complex answers ordered smallest to largest real part, then smallest to largest imaginary part.)
x= ?? multiplicty= ??
x= ?? multiplicty= ??
x= ?? multiplicty= ??
x= ?? multiplicty= ??
Answers
Answer: (x^2-1)(x^2+1)=(x-1)(x+1)(x^2+1)
Step-by-step explanation:
(x²+1)(x²-1) are factors of polynomial x4-1 and x=1,-1, i are the roots of x⁴ − 1.
What is Polynomial?A polynomial is an expression which is composed of variables, constants and exponents, that are combined using mathematical operations such as addition, subtraction, multiplication and division.
The given polynomial is Q(x) = x⁴ − 1.
We can write it as (x²)²-1²
We have a algebraic formula which is
a²-b²=(a+b)(a-b)
Similarly
(x²)²-1²=(x²+1)(x²-1)
Now let us find the roots of factors (x²+1) and (x²-1)
x²+1=x=√-1=i,-i
x²-1=x=1,-1
The multiplicity of the roots is always one.
Hence (x²+1)(x²-1) are factors of polynomial x4-1 and x=1,-1, i are the roots of x⁴ − 1.
To learn more on Polynomials click:
https://brainly.com/question/11536910
#SPJ2
What is the volume of the cylinder below? Use the formula V = πr²h
Answers
Answer:
[tex]\boxed{V = 339.12 ft^3}[/tex]
Step-by-step explanation:
V = [tex]\pi r^2 h[/tex]
Where r = 3, h = 12
V = (3.14)(3)²(12)
V = (3.14)(9)(12)
V = 339.12 ft³
what is the slope of the line with the equation y= -2x - 1? A -2 B -1 C 2 D 1
Answers
Answer:
The answer is "A'' -2 because anything next to the letter x is the slope
Step-by-step explanation:
The equation to slops is y=mx+b
m= slope
b= y intercept
Slope = -2
Y intercept = ( 0 , -1)
Step-by-step explanation:
Hope this helps. Your answer would be A
Construct a frequency distribution and a frequency histogram for the given data set using the indicated number of classes. Describe any patterns.
Number of classes: 8
Data set: Reaction times (in milliseconds) of 30 adult females to an auditory stimulus.
430 386 352 301 450 291 429 467 454 385 380
373 386 307 321 336 310 413 306 357 514 443
442 326 508 424 386 429 412 418
Answers
Answer:
The histogram for the data is attached below.
Step-by-step explanation:
Arrange the data in ascending order as follows:
S = {291 , 301 , 306 , 307 , 310 , 321 , 326 , 336 , 352 , 357 , 373 , 380 , 385 , 386 , 386 , 386 , 412 , 413 , 418 , 424 , 429 , 429 , 430 , 442 , 443 , 450 , 454 , 467 , 508 , 514}
Compute the range:
[tex]Range=Max.-Min.\\=514-291\\=223[/tex]
Compute the class width:
[tex]Class\ Width =\frac{Range}{No.\ of\ classes}=\frac{223}{8}=27.875\approx 28[/tex]
The classes are as follows:
290 - 318
319 - 347
348 - 376
377 - 405
406 - 434
435 - 463
464 - 492
493 - 521
Compute the frequency distribution as follows:
Class Interval Frequency
290 - 318 5
319 - 347 3
348 - 376 3
377 - 405 5
406 - 434 7
435 - 463 4
464 - 492 1
493 - 521 2
The histogram for the data is attached below.
Regulation baseballs have a diameter that is either 23.2 mm or 24.2 mm. What is the difference in volume of the baseballs? Round to the nearest hundredth. Use pi equals 3.14. V equals ____________ mm cubed Type your numerical answer (without units) below.
Answers
Answer:
ΔV = 865.51 mm^3
Step-by-step explanation:
In order to calculate the difference in volume between both baseballs you use the following formula for the volume of a sphere:
[tex]V=\frac{4}{3}\pi r^3[/tex] (1)
where r is the radius of he sphere.
You calculate the volume of each sphere:
First baseball:
radius = 23.2mm/2 = 11.61mm
[tex]V_1=\frac{4}{3}\pi (11.61mm)^3=6555.18\ mm^3[/tex]
Second baseball:
radius = 24.2mm/2 = 12.1mm
[tex]V_2=\frac{4}{3}\pi (12.10)^3=7420.70\ mm^3[/tex]
Then, the difference in the volumen of both spheres is:
[tex]\Delta V=V_2-V_1=7420\ mm^3-6555.18\ mm^3=865.51\ mm^3[/tex]
Ingredients
•1 1/2
cups all-purpose flour.
• 31/2 teaspoons baking powder.
• 1 teaspoon salt.
1 tablespoon white sugar.
• 1 1/4 cups milk.
• 1 egg.
• 3 tablespoons butter.
1.) How much of each of the ingredients do you need to make 16 pancakes, 4 pancakes, 12pancakes? Explain which operations with fractions you used to obtain your answer.
Answers
Answer:
To make 16 pancakes, we would multiply each of the initial measurement of the ingredients by 16.
To make 4 pancakes, we would multiply each of the initial measurement of the ingredients by 4.
To make 12 pancakes, we would multiply each of the initial measurement of the ingredients by 12.
see explanation below
Step-by-step explanation:
If the ingredients to make 1 pancake is as follows:
1 1/2 cups all-purpose flour; 31/2 teaspoons baking powder; 1 teaspoon salt.
1 tablespoon white sugar.; 1 1/4 cups milk; 1 egg; and 3 tablespoons butter.
Then to make 16 pancakes, we would multiply each of the initial measurement of the ingredients by 16.
cups all-purpose flour =16× 1 1/2
= 16×3/2 = 24
teaspoons baking powder = 16 ×7/2 = 56
teaspoon salt = 1×16 = 16
tablespoon white sugar= 1×16 = 16
cups milk= 16×5/4 = 20
egg= 1×16 = 16
tablespoons butter= 3×16 = 48
Then to make 4 pancakes, we would multiply each of the initial measurement of the ingredients by 4.
cups all-purpose flour =4× 1 1/2
= 4×3/2 = 6
teaspoons baking powder = 4 ×7/2 =14
teaspoon salt = 1×4 = 4
tablespoon white sugar= 1×4 = 4
cups milk= 4×5/4 = 5
egg= 1×4 = 4
tablespoons butter= 3×4 = 12
Then to make 12 pancakes, we would multiply each of the initial measurement of the ingredients by 12.
cups all-purpose flour =12× 1 1/2
= 12×3/2 = 18
teaspoons baking powder = 12 ×7/2 =42
teaspoon salt = 1×12 = 12
tablespoon white sugar= 1×12 = 12
cups milk= 12×5/4 = 15
egg= 1×12 = 12
tablespoons butter= 3×12 = 36
The operation applied is multiplication with each fraction.
2. The half life of uranium-232 is 68.9 years. a) If you have a 100 gram sample, how much would be left after 250 years? b) If you have a 100 gram sample, how long would it take for there to be 12.5 grams remaining?
Answers
Step-by-step explanation:
The amount left is:
A = A₀ (½)^(t/T)
where A₀ is the initial amount,
t is the amount of time,
and T is the half life.
a) A = 100 g (½)^(250 yr / 68.9 yr)
A = 8.09 g
b) 12.5 g = 100 g (½)^(t / 68.9 yr)
0.125 = (½)^(t / 68.9 yr)
3 = t / 68.9 yr
t = 206.7 yr
Please answer soon. Include Statement and Reason if possible.
Given: ΔABC, AC = BC, AB = 3
CD ⊥ AB, CD = √3
Find: AC
Answers
====================================================
Explanation:
Triangle CDA is congruent to triangle CDB. We can use the HL (hypotenuse leg) congruence theorem to prove this. This only works because we have two right triangles.
Since CDA and CDB are congruent, this means their corresponding pieces are the same length. Specifically AD = DB, so
AD+DB = AB
AD+AD = 3
2*AD = 3
AD = 3/2 = 1.5
For triangle CDA, we have AD = 3/2 = 1.5 and CD = sqrt(3). We can use the pythagorean theorem to find the missing side AC
a^2 + b^2 = c^2
(AD)^2 + (CD)^2 = (AC)^2
(3/2)^2 + (sqrt(3))^2 = (AC)^2
9/4 + 3 = (AC)^2
(AC)^2 = 9/4 + 3
(AC)^2 = 9/4 + 12/4
(AC)^2 = 21/4
AC = sqrt(21/4)
AC = sqrt(21)/sqrt(4)
AC = sqrt(21)/2
This is the same as writing (1/2)*sqrt(21) or 0.5*sqrt(21)
The area of a square is 64n36. What is the length of one side of the square?
Answers
Answer:
8n6
step by step explanation.
45% of 80.374 is a number between
Answers
Answer:
36.1683
Step-by-step explanation:
45*80.374/100=
The charge to rent a trailer is $2525 for up to 2 hours plus $99 per additional hour or portion of an hour. Find the cost to rent a trailer for 2.82.8 hours, 33 hours, and 8.78.7 hours. Then graph all ordered pairs, (hours, cost), for the function.
Answers
The question is not written properly! Complete question along with answer and step by step explanation is provided below.
Question:
The charge to rent a trailer is $25 for up to 2 hours plus $9 per additional hour or portion of an hour.
Find the cost to rent a trailer for 2.8 hours, 3 hours, and 8.7 hours.
Then graph all ordered pairs, (hours, cost), for the function.
Answer:
ordered pair = (2.8, 34)
ordered pair = (3, 34)
ordered pair = (8.7, 88)
Step-by-step explanation:
Charge for 2.8 hours:
$25 for 2 hours
$9 for 0.8 hour
Total = $25 + $9
Total = $34
ordered pair = (2.8, 34)
Charge for 3 hours:
$25 for 2 hours
$9 for 1 hour
Total = $25 + $9
Total = $34
ordered pair = (3, 34)
Charge for 8.7 hours:
$25 for 2 hours
$9 for 1 hour
$9 for 1 hour
$9 for 1 hour
$9 for 1 hour
$9 for 1 hour
$9 for 1 hour
$9 for 0.7 hour
Total = $25 + $9 + $9 + $9 + $9 + $9 + $9 + $9
Total = $88
ordered pair = (8.7, 88)
The obtained ordered pairs are graphed, please refer to the attached graph.
A horse is free to roam and feed in an enclosed pasture that is 150 feet by 200 feet. At any given time, what is the probability that the horse is at least 25 feet away from all boundary lines?
Answers
25/250 = 1/10
I do not know that much english. I an spanish so I might have done it wrong.
the probability that the horse is at least 25 feet away from all boundary lines is 0.5
To calculate the probability that the horse is at least 25 feet away from all boundary lines in an enclosed pasture that is 150 feet by 200 feet, we need to consider the available space for the horse to roam.
The area of the pasture is given by:
Area = Length * Width = 150 feet * 200 feet = 30,000 square feet
Now, let's consider the area where the horse must stay within at least 25 feet away from all boundary lines. We can imagine this as a rectangular strip along the inner boundary of the pasture, with dimensions reduced by 50 feet on each side.
The dimensions of this rectangular strip would be:
Length = 150 feet - 2 * 25 feet = 100 feet
Width = 200 feet - 2 * 25 feet = 150 feet
The area of this rectangular strip is given by:
Area_strip = Length * Width = 100 feet * 150 feet = 15,000 square feet
Therefore, the probability that the horse is at least 25 feet away from all boundary lines is:
Probability = Area_strip / Area = 15,000 square feet / 30,000 square feet = 0.5
The probability is 0.5 or 50%.
Learn more about probability here
https://brainly.com/question/32117953
#SPJ2
Question
Given that tan(0) =5/12
and 0 is in Quadrant III. what is cos(0)? Write your answer in exact form. Do not round.
Provide your answer below:
Answers
Answer:
cosΘ = - [tex]\frac{12}{13}[/tex]
Step-by-step explanation:
Given that Θ is in the third quadrant then cosΘ < 0
Given
tanΘ = [tex]\frac{5}{12}[/tex] = [tex]\frac{opposite}{adjacent}[/tex]
Then 5 and 12 are the legs of a right triangle (5- 12- 13 ) with hypotenuse = 13
Thus
cosΘ = - [tex]\frac{adjacent}{hypotenuse}[/tex] = - [tex]\frac{12}{13}[/tex]
√9m^2n^2 + 2√m^2n^2 - 3mn
Answers
Answer:
I think it is
Step-by-step explanation:
Answer:
5n√2m^ - 3mn
Step-by-step explanation:
solve. 5 (y-1)+6= -9
Answers
5y-6 + 6= -9
5y=-9
y=-9/5 or -1.8
Answer:
y=-2
Step-by-step explanation:
5 (y-1)+6= -9
Subtract 6 from each side
5 (y-1)+6-6= -9-6
5 ( y-1) = -15
Divide by 5
5(y-1)/5 = -15/5
y-1 = -3
Add 1 to each side
y-1+1 = -3+1
y = -2
68. A hexagon has two sides each of length 3x inches. It has three sides each of length 2x inches. The sixth side has a length of 15 inches. If the perimeter of the hexagon is 135 inches, what is the value of x?
E.4
F.5
G. l0
H. 15
Answers
Answer:
G 10
Step-by-step explanation:
2*3x+3*2x+15=135 inches
6x+6x=120 inches
12x=120 inches
x= 10 inches
∛3375-[tex]\sqrt[4]{38416}[/tex]=?
Answers
Answer:
1
Step-by-step explanation:
=> [tex]\sqrt[3]{3375} - \sqrt[4]{38416}[/tex]
Factorizing 3375 gives 15 * 15 * 15 which equals 15^3 and factorizing 38416 gives 14 * 14 * 14 * 14 which equals 14^4
=> [tex]\sqrt[3]{15^3} - \sqrt[4]{14^4}[/tex]
=> 15 - 14
=> 1
Answer:
1Step-by-step explanation:
[tex] \sqrt[3]{3375} - \sqrt[4]{38416} [/tex]
Calculate the cube root
[tex] \sqrt[3]{ {15}^{3} } - \sqrt[4]{38416} [/tex]
Calculate the root
[tex] \sqrt[3]{ {15}^{3} } - \sqrt[4]{ {14}^{4} } [/tex]
[tex] {15}^{ \frac{3}{3} } - {14}^{ \frac{4}{4} } [/tex]
[tex]15 - 14[/tex]
Subtract the numbers
[tex]1[/tex]
Hope this helps...
The area of the region under the curve of the function f(x)=5x+7 on the interval [1,b] is 88 square units, where b>1. What is the value of b.
Answers
Answer:
[tex]\displaystyle b = 5[/tex]
General Formulas and Concepts:
Calculus
Integration
IntegralsIntegration Rule [Reverse Power Rule]: [tex]\displaystyle \int {x^n} \, dx = \frac{x^{n + 1}}{n + 1} + C[/tex]
Integration Rule [Fundamental Theorem of Calculus 1]: [tex]\displaystyle \int\limits^b_a {f(x)} \, dx = F(b) - F(a)[/tex]
Integration Property [Multiplied Constant]: [tex]\displaystyle \int {cf(x)} \, dx = c \int {f(x)} \, dx[/tex]
Integration Property [Addition/Subtraction]: [tex]\displaystyle \int {[f(x) \pm g(x)]} \, dx = \int {f(x)} \, dx \pm \int {g(x)} \, dx[/tex]
Area of a Region Formula: [tex]\displaystyle A = \int\limits^b_a {[f(x) - g(x)]} \, dx[/tex]
Step-by-step explanation:
Step 1: Define
Identify
[tex]\displaystyle \int\limits^b_1 {5x + 7} \, dx = 88[/tex]
Step 2: Solve
[Integral] Rewrite [Integration Property - Addition/Subtraction]: [tex]\displaystyle \int\limits^b_1 {5x} \, dx + \int\limits^b_1 {7} \, dx = 88[/tex][Integrals] Rewrite [Integration Property - Multiplied Constant]: [tex]\displaystyle 5 \int\limits^b_1 {x} \, dx + 7 \int\limits^b_1 {} \, dx = 88[/tex][Integrals] Integration Rule [Reverse Power Rule]: [tex]\displaystyle 5 \bigg( \frac{x^2}{2} \bigg) \bigg| \limits^b_1 + 7(x) \bigg| \limits^b_1 = 88[/tex]Evaluate [Integration Rule - Fundamental Theorem of Calculus 1]: [tex]\displaystyle 5 \bigg( \frac{b^2}{2} - \frac{1}{2} \bigg) + 7(b - 1) = 88[/tex]Simplify: [tex]\displaystyle \frac{5b^2}{2} - \frac{5}{2} + 7b - 7 = 88[/tex]Isolate: [tex]\displaystyle \frac{5b^2}{2} + 7b = \frac{195}{2}[/tex]Solve: [tex]\displaystyle b = \frac{-39}{5} ,\ 5[/tex]Topic: AP Calculus AB/BC (Calculus I/I + II)
Unit: Integration
Answer:5
Step-by-step explanation:
got it right
Line B passes through the points (5, 10) and (0, 0). What is the slope of the line perpendicular to line B?
Answers
Answer:
The line that is perpendicular to B has a slope of -1/2
Step-by-step explanation:
First find the slope of line B
m = ( y2-y1)/(x2-x1)
= (10-0)/(5-0)
= 10/5
= 2
Lines that are perpendicular have slopes that multiply to -1
m * 2 = -1
m = -1/2
The line that is perpendicular to B has a slope of -1/2
Answer:
-1/2x
Step-by-step explanation:
Hey there! :)
Well to find the slope of line B we'll use the following formula.
[tex]\frac{y_{2} - y_{1} }{x_{2} - x_{1} }[/tex]
We'll use the points (0,0) and (5,10),
10 - 0 = 10
5 - 0 = 5
Slope = 2x
The slopes of 2 perpendicular lines are reciprocals of each other,
meaning if the slope of line B is 2x then its perpendicular lines slope is -1/2x.
Hope this helps :)